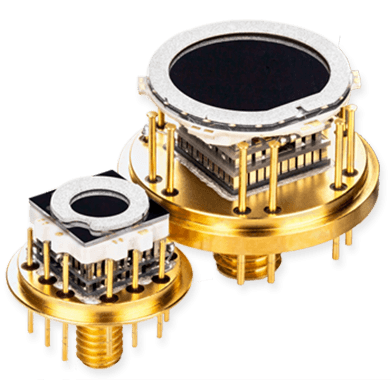
KETEK is the world’s leading manufacturer of Silicon Drift Detectors, which are used in an almost infinite number of industrial material analysis applications. Our SDDs can be found in X-ray fluorescence spectrometers & electron microscopes and contribute to countless material research experiments around the Earth – and some even on Mars.
Welcome to KETEK.
16Oct 2023
India’s Chandrayaan-3 moon rover equipped with KETEK’s VITUS H30 SDD detects minor elements
The Alpha Particle X-ray Spectrometer (APXS) with a KETEK VITUS H30 SDD onboard the Indian Chandrayaan-3 moon rover has discovered the presence of interesting minor elements, including Sulfur, apart from the major expected [...]